Law of Gearing

Condition for Constant Velocity Ratio of Toothed Wheels – Law of Gearing

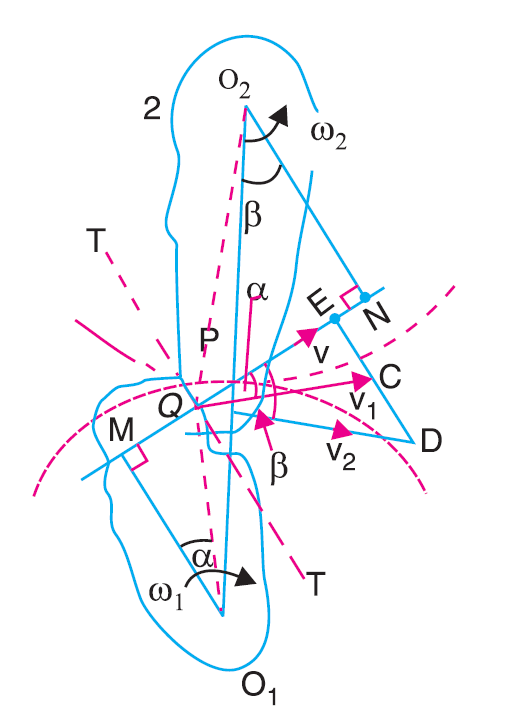
Consider the portions of the two teeth, one on the wheel 1 (or pinion) and the other on the wheel 2. Let the two teeth come in contact at point Q, and the wheels rotate in the directions as shown in the figure.

Let T T be the common tangent and MN be the common normal to the curves at the point of contact Q. From the centres O₁ and O₂, draw O₁M and O₂N perpendicular to MN. A little consideration will show that the point Q moves in the direction QC, when considered as a point on wheel 1, and in the direction QD when considered as a point on wheel 2.

Let v₁ and v₂ be the velocities of the point Q on the wheels 1 and 2 respectively. If the teeth are to remain in contact, then the components of these velocities along the common normal MN must be equal.

$v_{1}\cos \alpha =v_{2}\cos \beta$ $(\omega _{1}\times O_{1}Q)\cos \alpha =(\omega _{2}\times O_{2}Q)\cos \beta$ $(\omega _{1}\times O_{1}Q)\frac{O_{1}M}{O_{2}Q} =(\omega _{2}\times O_{2}Q)\frac{O_{2}N}{O_{2}Q}$ $\omega _{1}\times O_{1}M =\omega _{2}\times O_{2}N$ $\frac{\omega _{1}}{\omega _{2}}=\frac{O_{2}N}{O_{1}M}$

Also from similar triangles O₁MP and O₂NP
$\frac{O_{2}N}{O_{1}M}=\frac{O_{2}P}{O_{1}P}$ Combining equations we have
$\frac{\omega _{1}}{\omega _{2}}=\frac{O_{2}N}{O_{1}M}=\frac{O_{2}P}{O_{1}P}$

From above, we see that the angular velocity ratio is inversely proportional to the ratio of the distances of the point P from the centers O₁ and O₂, or the common normal to the two surfaces at the point of contact Q intersects the line of centers at point P which divides the center distance inversely as the ratio of angular velocities.

Therefore in order to have a constant angular velocity ratio for all positions of the wheels, the point P must be the fixed point (called pitch point) for the two wheels. In other words, the common normal at the point of contact between a pair of teeth must always pass through the pitch point. This is the fundamental condition which must be satisfied while designing the profiles for the teeth of gear wheels. It is also known as law of gearing.

Notes:

The above condition is fulfilled by teeth of involute form, provided that the root circles from which the profiles are generated are tangential to the common normal.
If the shape of one tooth profile is arbitrarily chosen and another tooth is designed to satisfy the above condition, then the second tooth is said to be conjugate to the first. The conjugate teeth are not in common use because of difficulty in manufacture, and cost of production.
If D₁ and D₂ are pitch circle diameters of wheels 1 and 2 having teeth T₁ and T₂ respectively, then velocity ratio,

$\frac{\omega _{1}}{\omega _{2}}=\frac{O_{2}P}{O_{1}P}=\frac{D_{1}}{D_{2}}=\frac{T_{1}}{T_{2}}$

Velocity of Sliding of Teeth

The sliding between a pair of teeth in contact at Q occurs along the common tangent T T to the tooth curves. The velocity of sliding is the velocity of one tooth relative to its mating tooth along the common tangent at the point of contact.

The velocity of point Q, considered as a point on wheel 1, along the common tangent T T is represented by EC. From similar triangles QEC and O₁MQ,
$\frac{EC}{MQ}=\frac{v_{1}}{O_{1}Q}=\omega _{1}$ or $EC=\omega _{1}.MQ$

Similarly, the velocity of point Q, considered as a point on wheel 2, along the common tangent T T is represented by ED. From similar triangles QCD and O₂NQ,

$\frac{ED}{QN}=\frac{v_{2}}{O_{2}Q}=\omega _{2}$ or $ED=\omega _{2}.QN$

Let v_s = Velocity of sliding at Q.

$v_{s}=ED-EC=\omega _{2}.QN-\omega _{1}.MQ$ $v_{s}=\omega _{2}(QP+PN)-\omega _{1}(MP-QP)$
$v_{s}=(\omega _{1}+\omega _{2})QP+\omega _{2}.PN-\omega _{1}MP$ ..(i)
Since $\frac{\omega _{1}}{\omega _{2}}=\frac{O_{2}P}{O_{1}P}=\frac{PN}{NP}$ or $\omega _{1}.MP=\omega _{2}.PN$
Therefore the equation becomes $v_{s}=(\omega _{1}+\omega _{2})QP$ ..(ii)

Notes:

We see from equation (ii), that the velocity of sliding is proportional to the distance of the point of contact from the pitch point.
Since the angular velocity of wheel 2 relative to wheel 1 is and P is the instantaneous center for this relative motion, therefore the value of v_s may directly be written as v_s QP, without the above analysis.

References:

Khurmi, R. et al., Figure 12.6 Theory of Machines, 14th ed.

For curious minds, here’s an excellent video explaining how a gearbox works:

https://www.youtube.com/watch?v=VrhRGbmJW2s

Back To Top

Next Page: Simple Gear Selection Procedure

Previous Page: Gear Nomenclature

Recent Posts